
Snowflake macro photography 1 (c) Alexey Kljatov
(CC BY-SA 4.0)
Since the start of January, I have expected snow to come because the first snow is usually around this time period. However, it is already halfway through January and rain- snow is the closest I have seen. While looking at a paper decoration of the snowflake model, I had noticed some self – similarity and difference at the same time. Then a question popped into my head: Is snowflake a fractal?
What is a fractal?
Fractal is a geometric pattern which is self – similar and the pattern continues on forever. So if you zoom in to a part of the pattern, it will be a “smaller” version of its original self. Also, another trait of the fractal is a non – integer dimension. Dimension is the amount of axis it has. For example a dot has 0 dimension, a line has 1 dimension(length), a shape has 2 dimension(length*width), an object has 3 dimension (length*width*height). All of the examples are integer dimension. Depending on the type of fractal, the dimension will vary such as dragon curves(1-2 D) because of a “shape” composed by lines and landscape images(2-3 D) because some part of it has the “texture” which is more inclined towards 3D.
Yes or no
Depending on the type of snowflake, the answer is going to be different.
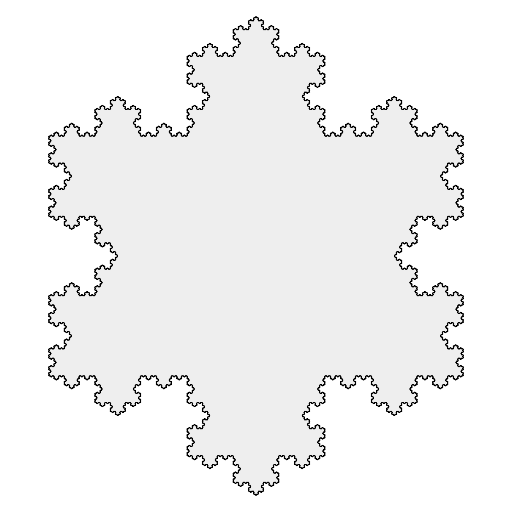
Koch Snowflake 7th iteration (c) Wrtlprnft
CC0
There is a famous fractal pattern called the Koch snowflake. It is a fractal because it has the pattern of dividing a side into 3 equal segments and draw an equilateral triangle in the center segment. This way when you “zoom in” to each side it has the same pattern. However, Koch snowflake isn’t a real snowflake. In a real snowflake, each of the branches contains some self- similarity but the pattern doesn’t continue on forever. So it isn’t a fractal but it has fractal geometry in it.
Conclusion
Snowflake isn’t a fractal because it has a limit to how many times itself repeats and every snowflake is slightly different from each other. Since all of the main branches are self – similar to another, it has the fractal component. Also, a fractal model snowflake can have a 95% or 99% similar to an actual snowflake. However, the Koch snowflake is a fractal but it isn’t a tangible snowflake.
Leave a Reply